Coordinate Geometry Class 10 Important Questions | NCERT Maths Chapter-7
Coordinate Geometry Class 10 Important Questions
The start of class 10 marks the beginning of the foundation for class 11 and class 12. It is very important study the basics in order to understand each and every chapter properly. In this page, we have provided all the important question for cbse class 9 that could be asked in the examination. Students also need to study the ncert solutions for class 10 in order to gain more knowledge and understanding the lessons. Questions and Answers are way to learn the new things in a proper way. NCERT textbooks downloads for class 9 in pdf are also available for the students if they need more help. By downloading these books, they can study from it. Our experts also prepared revision notes for class 9 so that students should see the details of each and every chapters. Class 9 important questions are the best to revise all the chapters in the best way.
Question 1.
Find the value of ‘k”, for which the points are collinear: (7, -2), (5, 1), (3, k).
Solution:
Let the given points be
A (x1, y1) = (7, -2), B (x2, Y2) = (5, 1) and C (x3, y3) = (3, k)
Since these points are collinear therefore area (∆ABC) = 0
⇒ \(\frac{1}{2}\) [x1(y2 – Y3) + x2(y3 – y1) + x3(y1 – y2)] = 0
⇒ x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2) = 0
⇒ 7(1 – k) + 5(k + 2) + 3(-2 -1) = 0
⇒ 7 – 7k + 5k + 10 – 9 = 0
⇒ -2k + 8 = 0
⇒ 2k = 8
⇒ k = 4
Hence, given points are collinear for k = 4.
Question 2.
Find the area of the triangle formed by joining the mid-points of the sides of the triangle whose vertices are (0, -1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle.
Solution:
Let A (x1, y1) = (0, -1), B (x2, y2) = (2, 1), C (x3, y3) = (0, 3) be the vertices of ∆ABC.
Now, let P, Q, R be the mid-points of BC, CA and AB, respectively.
So, coordinates of P, Q, R are
Ratio of ar (∆PQR) to the ar (∆ABC) = 1 : 4.
Question 3.
Find the area of the quadrilateral whose vertices, taken in order, are (-4, -2), (-3, -5), (3, -2) and (2, 3).
Solution:
Let A(4, -2), B(-3, -5), C(3, -2) and D(2, 3) be the vertices of the quadrilateral ABCD.
Now, area of quadrilateral ABCD
= area of ∆ABC + area of ∆ADC

Question 4.
A median of a triangle divides it into two triangles of equal areas. Verify this result for ∆ABC whose vertices are A (4,-6), B (3, -2) and C (5, 2).
Solution:
Since AD is the median of ∆ABC, therefore, D is the mid-point of BC.

Hence, the median divides it into two triangles of equal areas.
Question 5.
Find the ratio in which the point P (x, 2), divides the line segment joining the points A (12, 5) and B (4, -3). Also find the value of x.
Solution:
The ratio in which p divides the line segment is \(\frac{3}{5}\), i.e., 3 : 5.
Question 6.
If A (4, 2), B (7, 6) and C (1, 4) are the vertices of a ∆ABC and AD is its median, prove that the median AD divides into two triangles of equal areas.
Solution:
Given: AD is the median on BC.
⇒ BD = DC
The coordinates of midpoint D are given by.
Hence, AD divides ∆ABC into two equal areas.
Question 7.
If the point A (2, -4) is equidistant from P (3, 8) and Q (-10, y), find the values of y. Also find distance PQ.
Solution:
Given points are A(2, 4), P(3, 8) and Q(-10, y)
According to the question,
PA = QA
Question 8.
The base BC of an equilateral triangle ABC lies on y-axis. The coordinates of point Care (0, -3). The origin is the mid-point of the base. Find the coordinates of the points A and B. Also find the coordinates of another point D such that BACD is a rhombus.
Solution:
∵ O is the mid-point of the base BC.
∴ Coordinates of point B are (0, 3). So,
BC = 6 units Let the coordinates of point A be (x, 0).
Using distance formula,

∴ Coordinates of point A = (x, 0) = (3√3, 0)
Since BACD is a rhombus.
∴ AB = AC = CD = DB
∴ Coordinates of point D = (-3√3, 0).
Question 9.
Prove that the area of a triangle with vertices (t, t-2), (t + 2, t + 2) and (t + 3, t) is independent of t.
Solution:
Area of a triangle = \(\frac{1}{2}\) [x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
Area of the triangle = \(\frac{1}{2}\)[t + 2 – t) + (t + 2) (t – t + 2) + (t + 3) (t – 2 – t – 2)]
= \(\frac{1}{2}\) [2t + 2t + 4 – 4t – 12 ]
= 4 sq. units
which is independent of t.
Hence proved.
Question 10.
The area of a triangle is 5 sq units. Two of its vertices are (2, 1) and (3, -2). If the third vertex is (\(\frac{7}{2}\), y), find the value of y.
Solution:
Given: ar(∆ABC) = 5 sq. units

Question 11.
The coordinates of the points A, B and Care (6, 3), (-3,5) and (4,-2) respectively. P(x, y) is any point in the plane. Show that \(\frac { ar(∆PBC) }{ ar(∆ABC) } \) = \(\frac{x+y-2}{7}\)
Solution:
P(x, y), B(-3, 5), C(4, -2), A(6, 3)

Question 12.
In Fig. 6.32, the vertices of ∆ABC are A(4, 6), B(1, 5) and C(7, 2). A line-segment DE is drawn to intersect the sides AB and AC at D and E respectively such that \(\frac{A D}{A B}=\frac{A E}{A C}=\frac{1}{3}\) Calculate the area of ∆ADE and compare it with area of ∆ABC.
Solution:
\
Question 13.
If a = b = 0, prove that the points (a, a2), (b, b2) (0, 0) will not be collinear.
Solution:
∵ We know that three points are collinear if area of triangle = 0
∴ Area of triangle with vertices (a, a2), (b, b2) and (0, 0)

∵ Area of ∆ ≠ 0
∴ Given points are not collinear.
Coordinate Geometry Class 10 Extra Questions HOTS
Question 1.
The line joining the points (2, 1) and (5, -8) is trisected by the points P and Q. If the point P lies on the line 2x – y + k = 0, find the value of k.
Solution:

As line segment AB is trisected by the points P and Q.
Therefore,
Case I: When AP : PB = 1 : 2.

⇒ P (3, -2)
Since the point P (3,-2) lies on the line
2x – y + k = 0 =
⇒ 2 × 3-(-2) + k = 0
⇒ k = -8
Case II: When AP : PB = 2 : 1.

Coordinates of point P are![]()
Since the point P(4, -5) lies on the line
2x – y + k = 0
∴ 2 × 4-(-5) + k = 0
∴ k = -13
Question 2.
Prove that the diagonals of a rectangle bisect each other and are equal.
Solution:
Let OACB be a rectangle such that OA is along x-axis and OB is along y-axis. Let OA = a and OB = b.
Then, the coordinates of A and B are (a,0) and (0, b) respectively.
Since, OACB is a rectangle. Therefore,
AC = OB
⇒ AC = b
Also, OA = a
⇒ BC = a
So, the coordinates of Care (a, b).
∴ OC = AB.
Question 3.
In what ratio does the y-axis divide the line segment joining the point P (4, 5) and Q (3, -7)?
Also, find the coordinates of the point of intersection.
Solution:
Suppose y-axis divides PQ in the ratio k : 1. Then, the coordinates of the point of division are|
Question 4.
Find the centre of a circle passing through the points (6, -6), (3, -7) and (3, 3).
Solution:
Let O(x, y) be the centre of circle. Given points are A(6, -6), B(3, -7) and C(3, 3).
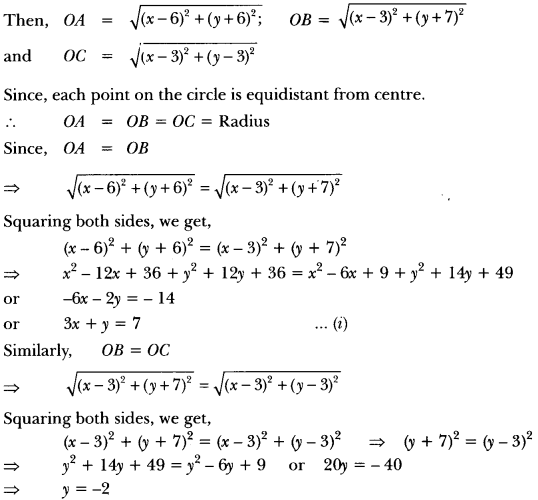
Question 5.
If the coordinates of the mid-points of the sides of a triangle are (1, 1), (2, – 3) and (3, 4). Find its centroid.
Solution:
Let P(1, 1), Q(2, -3), R(3, 4) be the mid-points of sides AB, BC and CA respectively, of triangle ABC. Let A(x1, y1), B(x2, y2) and C(x3, y3) be the vertices of triangle ABC. Then, P is the mid-point of AB.
Question 6.
Determine the ratio in which the line 2x + y – 4 = 0 divides the line segment joining the points A(2, -2) and B(3, 7).
Solution:
Let P(x1, y1) be common point of both lines and divide the line segment joining A(2, -2) and B(3, 7) in ratio k : 1.
Question 7.
Show that ∆ABC with vertices A (-2, 0), B (2, 0) and C (0, 2) is similar to ∆DEF with vertices
D(4, 0) E (4, 0) and F (0, 4).
Solution:
Given vertices of ∆ABC and ∆DEF are
A(-2, 0), B(2, 0), C(0, 2), D(-4, 0), E(4, 0) and F(0, 4)![]()

Here, we see that sides of ∆DEF are twice the sides of a ∆ABC.
Hence, both triangles are similar.
Important Links
NCERT Quick Revision Notes- Coordinate Geometry
NCERT Solution- Coordinate Geometry
Important MCQs- Coordinate Geometry
For Free, Video Lectures Click here
Discover more from EduGrown School
Subscribe to get the latest posts sent to your email.
