Chapter-5 Understanding Elementary Shapes | Class 6th | NCERT MAths Solutions | Edugrown
Chapter-5 Understanding Elementary Shapes | Class 6th | NCERT MAths Solutions | Edugrown
NCERT Solutions for Class 6 Maths: One of the most important and interesting things to keep in mind is that these solutions available are totally free of cost. This also includes all the solutions to the exercises that are given in the textbook. NCERT solutions for class 6 maths gives you chapter-wise solutions to each and every question. This can help you solve even the tougher with ease.
It is always good to have a strong foundation in order to build a good building. This also is true in class when you are still learning the basics of maths. NCERT solutions for class 6 maths provides you the exact opportunity to build a strong foundation in this subject. Below is an overview of each and every chapter covered in the NCERT textbook.
NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes
Ex 5.1 Class 6 Maths Question 1.
What is the disadvantage in comparing line segments by mere observation?
Solution:
The disadvantage in comparing line segments by mere observation is the inaccuracy in judgement.
Ex 5.1 Class 6 Maths Question 2.
Why is it better to use a divider than a ruler, while measuring the length of a line segment?
Solution:
It is better to use a divider with ruler as it given an accurate measurement of the line segment.
Ex 5.1 Class 6 Maths Question 3.
Draw any line segment, say AB. Take any point C lying in between A and B. Measure the lengths of AB, BC and AC. Is AB = AC + CB?
[Note: If A, B, C are any three points on a line, such that AC + CB = AB, then we can be sure that C lies between A and B.]
Solution:
On measuring the lengths of line segments AB, BC and AC using ruler, we find![]()
AB =6.5 cm,
CB = 4.2 cm,
and AC = 2.3 cm
Now, AC +CB = 2.3 cm +4.2 cm = 6.5 cm = AB
AB = AC+CB.
Ex 5.1 Class 6 Maths Question 4.
If A, B, C are three points on a line such that AB = 5 cm, BC = 3 cm and AC = 8 cm, which one of them lies between the other two?
Solution:
Since, AB + BC = 5 cm +3 cm = 8 cm = AC
∴ A, B, C are collinear and B lies between A and C.
Ex 5.1 Class 6 Maths Question 5.
Verify, whether D is the mid point of AG¯¯¯¯¯¯¯¯.
Solution:
Since AD = AB +BC + CD = 3 units
and, DG = DE +EF + FG = 3 units
∵ AD = DG [∵ Each = 3 units]
Thus, D is the mid-point of AG¯¯¯¯¯¯¯¯.
Ex 5.1 Class 6 Maths Question 6.
If B is the mid point of AC¯¯¯¯¯¯¯¯ and C is the mid point of BD¯¯¯¯¯¯¯¯, where A, B, C, D lie on a straight line, say why AB = CD?
Solution:
∵ B is the mid point of AC¯¯¯¯¯¯¯¯. Therefore, AB = BC.
∵ C is the mid-point of BD¯¯¯¯¯¯¯¯. Therefore, BC = CD.
Thus, AB =BC = CD i.e., AB = CD.
Ex 5.1 Class 6 Maths Question 7.
Draw five triangles and measure their sides. Check in each case, if the sum of the lengths of any two sides is always less than the third side.
Solution:
Draw any five triangles, T1, T2, T3, T4 and T5. label each one as ∆ ABC. Measure, in each case, the three sides a = BC, b = CA and c = AB.
Let us tabulate the measurements of sides as under:
From the above table, we find that
(i) each value of b + c – a is positive;
(ii) each value of c + a – b is positive;
(iii) each value of a + b – c is positive.
Now, b + c – a is positive ⇒ b + c – a > 0 ⇒ b + c > a
c + a – b is positive ⇒ c + a- b > 0 ⇒ c + a > b
a+ b – c is positive ⇒ a + b – c > 0 ⇒ a + b > c
Thus, it is verified that the sum of any two sides of a triangle is greater than the third side.
∴ The statement ‘the sum of two sides is ever less than the third side’ is never true.
Ex 5.2 Class 6 Maths Question 1.
What fraction of a clockwise revolution does the hour hand of a clock turn through, when it goes from
(a) 3 to 9
(b) 4 to 7
(c) 7 to 10
(d) 12 to 9
(e) 1 to 10
(f) 6 to 3
Solution:
(a) 12 of a revolution
(b) 14 of a revolution
(c) 14 of a revolution
(d) 34 of a revolution
(e) 34 of a revolution
(f) 34 of a revolution
Ex 5.2 Class 6 Maths Question 2.
Where will the hand of a clock stop if it
(a) starts at 12 and makes 12 of a revolution, clockwise?
(b) starts at 2 and makes 12 of a revolution clockwise?
(c) starts at 5 and makes 14 of a revolution, clockwise?
(d) starts at 5 and makes 34 of a revolution, clockwise?
Solution:
(a) If the hand of clock starts at 12 and makes 12 of a revolution clockwise it reaches at 6.
(b) If the hand of clock starts at 2 and makes 12 of a revolution clockwise it reaches at 8.
(c) If the hand of clock starts at 5 and makes 14 of a revolution clockwise it reaches at 8.
(d) If the hand of clock starts at 5 and makes 34 of a revolution clockwise it reaches at 2.
Ex 5.2 Class 6 Maths Question 3.
Which direction will you face if you start facing
(a) east and make 12 of a revolution clockwise?
(b) east and make 112 of a revolution clockwise?
(c) west and make 34 of a revolution anti-clockwise?
(d) south and make one full revolution?
(Should we specify clockwise or anit-clockwise for this last question? Why nor?)
Solution:
(a) Facing east and on making 12 of a revolution clockwise, we will face west.
(b) Facing east and on making 112 of a revolution clockwise, we will face west.
(c) Facing west and on making 34 of a revolution anti-clockwise, we will face north.
(d) Facing south and on making 1 full revolution we will face south again.
There is no need to specify clockwise or anti-clockwise in the last question as turning by one full revolution (i.e., two straight angles) make us to reach the original position.
Ex 5.2 Class 6 Maths Question 4.
What part of a revolution have you turned through if you stand facing
(a) east and turn clockwise to face north?
(b) south and turn clockwise to face east?
(c) west and turn clockwise to face east?
Solution:
Figures drawn will dearly show the amount of revolution:
(a) 34 of a revolution
(b) 34 of a revolution
(c) 12 of a revolution
Ex 5.2 Class 6 Maths Question 5.
Find the number of right angles turned through by the hour hand of clock when it goes from
(a) 3 to 6
(b) 2 to 8
(c) 5 to 11
(d) 10 to 1
(e) 12 to 9
(f) 12 to 6
Solution:
Since turning by two straight angles (or four right angles) in the same direction makes a full turn. Thus for the movement of clock hand from 12 – 1,1 – 2, 2 – 3, … 11 – 12 i.e., 12 in number gives 4 right angles turn. Therefore,
(a) From 3 to 6 : 3 – 4, 4 – 5 and 5 – 6 i.e., 3 in number.
∴ Number of right angles turned through by the hour hand of a clock when it goes from 3 to 6
= (412×3) right angles = 1 right angles
(b) From 2 to 8 :2 – 3,3 – 4, 4 – 5, 5 – 6,6 – 7 and 7 – 8 i.e., 6 in number.
∴ Number of right angles turned through by the hour hand of a clock
when it goes from 2 to 8
= (412×6) right angles = 2 right angles
(c) From 5 to II : 5 – 6, 6 – 7, 7 – 8, 8 – 9, 9 – 10 and 10 – 11 i.e., 6 in number.
∴ Number of right angles turned through by the hour hand of a clock when it goes from 5 to 11
= (412×6) right angles = 2 right angles
(d) From 10 to 1 :10 – 11,11 -12 and 12 – 1 i.e., 3 in number.
∴ Number of right angles turned through by the hour hand of a clock when it goes from 10 to 1
= (412×3) right angles = 1 right angles
(e) From 12 to 9 : 12 – 1, 1 – 2, 2 – 3, 3 – 4, 4 – 5, 5 – 6, 6 – 7, 7 – 8 and 8 – 9 i.e., 9 in number
∴ Number of right angles turned through by the hour hand of a clock when it goes from 12 to 9
= (412×9) right angles = 1 right angles
(f) From 12 to- 6 : 12 – 1, 1 – 2, 2 – 3, 3 – 4, 4 – 5 and 5 – 6 i.e., 6 in number.
∴ Number of right angles turned through by the hour hand of a clock when it goes from 12 to 6
= (412×6) right angles = 2 right angles
Ex 5.2 Class 6 Maths Question 6.
How many right angles do you make if you start facing
(a) south and turn clockwise to west?
(b) north and turn anti-clockwise to east?
(c) west and turn to west?
(d) south and turn to north?
Solution:
Figures drawn will clearly indicate the number of right angles we make if we start facing:
(a) south and turn clockwise to west is 1 right angle.
(b) north and turn anti-clockwise to east are 3 right angles.
(c) west and turn to west are 4 right angles.
(d) south and turn to north are 2 right angles.
Ex 5.2 Class 6 Maths Question 7.
Where will the hour hand qfa clock stop if it starts
(a) from 6 and turns through 1 right angle?
(b) from 8 and turns through 2 right angles?
(c) from 10 and turns through 3 right angles?
(d) from 7 and turns through 2 straight angles?
Solution:
We know that in 12 hours hour hand turns through 4 right angles i.e., in a turn of 1 right angle, the hour hand moves 3 hours.
(a) If the hour hand of a clock starts from 6 and turns through 1 right angle then its hour hand stops at (6 +3) i.e., 9.
(b) If the hour hand of a clock starts from 8 and turns through 2 right
angles then its hour hand stops at (8 + 2 x 3) = 14 i.e., 2. .
(c) If the hour hand of a clock starts from 10 and turns through 3 right angles then its hour hand stops at (10+3×3) = 19 i.e., 7.
(d) If the hour hand of a clock starts from 7 and turns through 2 straight angles i.e., 4 right angles then its hour hand stops at (7 + 4 x 3) = 19 i.e., 7.
Ex 5.3 Class 6 Maths Question 1.
Match the following:
(i) Straight angle (a) Less than one-fourth of a revolution
(ii) Right angle (b) More than half a revolution
(iii) Acute angle (c) Half of a revolution
(iv) Obtuse angle (d) One-fourth of a revolution
(v) Reflex angle (e) Between 14 and 12 of a revolution
(f) One complete revolution
Solution:
On matching, we find
(i) → (c)
(ii) → (d)
(iii) → (a)
(iv) → (e)
(v) → (b)
Question 2.
Classify each one of the following angles as right, straight, acute, obtuse or reflex:
Solution:
Clearly, we find that
(a) → acute angle
(b) → obtuse angle
(c) → right angle
(d) → reflex angle
(e) → straight angle
(f) → acute angles.
Ex 5.4 Class 6 Maths Question 1.
What is the measure of
(i) a right angle?
(ii) a straight angle?
Solution:
(i) 1 right angle = 90°
(ii) 1 straight angle = 2 right angles = 2 x 90°=180°
Ex 5.4 Class 6 Maths Question 2.
Say True or False:
(a) The measure of an acute angle < 90°.
(b) The measure of an obtuse angle < 90°.
(c) The measure of a reflex angle > 180°.
(d) The measure of one complete revolution = 360°.
(e) If m∠A = 53° and m∠B = 35°, then m∠A > m∠B.
Solution:
(a) True
(b) False
(c) True
(d) True
(e) True
Ex 5.4 Class 6 Maths Question 3.
Write down the measures of
(a) some acute angles.
(b) some obtuse angles.
(give at least two examples of each).
Solution:
(a) Since the measure of an acute angle < 90°. So, two acute angles may be of 60° and 30°.
(b) Since the measure of an obtuse angle > 90°. So, two obtuse angles may be of 110° and 120°.
Ex 5.4 Class 6 Maths Question 4.
Measure the angles given below using the Protractor and write down the measure.
Solution:
On measuring the given angles using protractor, we find that
(a) 45°
(b) 125°
(c) 90°
(d) ∠1 = 40°, ∠2 = 125° and ∠3 = 95°.
Ex 5.4 Class 6 Maths Question 5.
Which angle has a large measure ? First estimate and then measure.
Measure of Angle A =
Measure of Angle B =
Solution:
Observing the given angles, we find that
∠B > ∠A
On measuring, we find that
Measure of Angle A =40°
Measure of Angle B = 65°
∴ ∠B > ∠A.
Ex 5.4 Class 6 Maths Question 6.
From these two angles which has larger measure? Estimate and then confirm by measuring them.
Solution:
On observing, we find that
∠B > ∠A
On measuring, we find that
∠A = 45° and ∠B =60°
Thus, ∠B > ∠A.
Ex 5.4 Class 6 Maths Question 7.
Fill in the blanks with acute, obtuse, right or straight:
(a) An angle whose measure is less than that of a right angle is ……. .
(b) An angle whose measure is greater than that of a right angle is ……… .
(c) An angle whose measure is the sum of the measures of two right angles is ……. .
(d) When the sum of the measures of two angles is that of a right angle, then each one of them is …… .
(e) When the sum of the measures of two angles is that of a straight angle and if one of them is acute then the other should be …… .
Solution:
(a) acute
(b) obtuse
(c) straight
(d) acute
(e) obtuse
Ex 5.4 Class 6 Maths Question 8.
Find the measure of the angle shown in each figure. (First estimate with your eyes and then find the actual measure with a protractor).
Solution:
On estimating without measuring, the measure of the angle shown in each figure is
(a) 45°
(b) 60°
(c) 125°
(d) 135°
On measuring with the protractor, we find their measures as under:
(a) 40°
(b) 65°
(c) 130°
(d) 135°
Ex 5.4 Class 6 Maths Question 9.
Find the angle measure between the hands of the clock in each figure:
Solution:
The angle measure between the hands of the clock in the time shown is as under:
At 9.00 a.m. : 90°
At 1.00 p.m. : 30°
At 6.00 p.m. : 180°
Ex 5.4 Class 6 Maths Question 10.
Investigate
In the given figure, the angle measures 30°. Look at the same figure through a magnifying glass. Does the angle becomes larger? Does the size of the angle change?
Solution:
Looking through a magnifying glass does not make the angle larger and so the size of the angle does not change.
Ex 5.4 Class 6 Maths Question 11.
Measure and classify each angle:
Solution:
The measure of angle and its classification is recorded in the given table as under:
Ex 5.5 Class 6 Maths Question 1.
Which of the following are models for perpendicular lines:
(a) The adjacent edges of a table top.
(b) The lines of a railway track.
(c) The line segments forming the letter ‘L’.
(d) The letter V.
Solution:
(a) Yes
(b) No
(c) Yes
(d) No
Ex 5.5 Class 6 Maths Question 2.
Let PQ¯¯¯¯¯¯¯¯ be the perpendicular to the line segment XY¯¯¯¯¯¯¯¯. Let PQ¯¯¯¯¯¯¯¯ and XY¯¯¯¯¯¯¯¯ intersect in the point A. What is the measure of ∠PAY?
Solution:
The measure of ∠PAY =90° [∵ PQ ⊥ XY, given]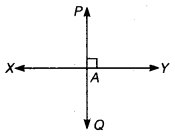
Ex 5.5 Class 6 Maths Question 3.
There are two “set-squares” in your box. What are the measures of the angles that are formed at their corners? Do they have any angle measure that is common?
Solution:
In one, the angles are of 30°, 60°, 90° and in the other the angles are of 90°, 45°, 45°.
Clearly, the common angle = 90°
Ex 5.5 Class 6 Maths Question 4.
Study the diagram. The line l is perpendicular to line m.
(a) Is CE = EG?
(b) Does PE bisect CG?
(c) Identify any two line segments for which PE is the perpendicular bisector. ‘ ’
(d) Are these true?
(i) AC > FG
(ii) CD = GH
(iii) BC< EH
Solution:
Ex 5.6 Class 6 Maths Question 1.
Name the types of following triangles:
(a) Triangle with lengths of sides 7 cm, 8 cm and 9 cm.
(b) ∆AJBC with AB = 8.7 cm, AC = 7 cm and BC = 6 cm.
(c) ∆PQR such that PQ = QR = PR = 5 cm.
(d) ∆DEF with m∠D = 90°
(e) ∆XYZ with m∠Y = 90° and XY = YZ.
(f) ∆LMN with m∠L = 30°, m∠M = 70° and m∠N = 80°
Solution:
(a) Since all the sides are of different lengths
∴ It is scalene triangle.
(b) In ∆ABC, AB ≠ BC ≠ CA.
∴ AABC is scalene triangle.
(c) In ∆PQR, PQ = QR = PR
∴ ∆PQR is equilateral triangle.
(d) In ∆DEF, m∠D =90°
∴ ∆DEF is right angled triangle.
(e) In ∆ XYZ, m∠Y = 90° and XY = YZ
∴ ∆ XYZ is an isosceles right angled.
(f) As each angle of ∆ LMN is < 90°
∴ ∆ LMN is an acute angled triangle.
Ex 5.6 Class 6 Maths Question 2.
Match the following:
Solution:
The matching is as shown:
(i) → (e)
(ii)→ (g)
(iii) → (a)
(iv) → (f)
(v) → (d)
(vi) → (c)
(vii) → (b)
Ex 5.6 Class 6 Maths Question 3.
Name each of the following triangles in two different ways: (you may judge the nature of the angle by observation)
Solution:
(a) Isosceles triangle; Acute angled triangle.
(b) Scalene triangle; Right angled triangle.
(c) Isosceles triangle; Obtuse angled triangle.
(d) Isosceles triangle; Right angled triangle.
(e) Equilateral triangle; Acute angled triangle.
(f) Scalene triangle; Obtuse angled triangle.
Ex 5.6 Class 6 Maths Question 4.
Try to construct triangles match sticks. Some are shown here. Gan you make a triangle with
(a) 3 matchsticks?
(b) 4 matchsticks?
(c) 5 matchsticks?
(d) 6 matchsticks?
(Remember you have to use all the available matchsticks in each case)
Name the type of triangle in each case.
If you cannot make a triangle, think of reasons for it.
Solution:
(a) Yes, an equilateral triangle.
(b) No (as sum of two sides is always > the third side but here 1 + 1 = 2 ≱ ≯ 2).
(c) Yes, an isosceles triangle.
(d) Yes, an equilateral triangle.
Ex 5.7 Class 6 Maths Question 1.
Say True or False:
(a) Each angle of a rectangle is a right angle.
(b) The opposite sides of a rectangle are equal in length.
(c) The diagonals of a square are perpendicular to ont i^Atber.
(d) All the sides of rhombus are of equal length.
(e) All the sides of a parallelogram are of equal length.
(f) The opposite sides of a trapezium are parallel.
Solution:
(a) True
(b) True
(c) True
(d) True
(e) False
(f) False
Ex 5.7 Class 6 Maths Question 2.
Give reasons for the following:
(a) A square can be thought of as a special rectangle.
(b) A rectangle can be thought of as a special parallelogram.
(c) A square can be thought of as a special rhombus.
(d) Squares, rectangles, parallelograms are all quadrilaterals.
(e) Square is also a parallelogram.
Solution:
(a) All the properties of a rectangle are there in a square.
(b) All the properties of a parallelogram are there in a rectangle.
(c) All the properties of a rhombus are there in a square.
(d) All the four sided closed plane figure are known as quadrilateral.
(e) All the properties of a parallelogram are there in a square.
Ex 5.7 Class 6 Maths Question 3.
A figure is said to be regular if its sides are equal in length and angles are equal in measure. Can you identify the regular quadrilateral?
Solution:
A regular quadrilateral is a square, regular pentagon, regular hexagon, regular octagon etc.
Ex 5.8 Class 6 Maths Question 1.
Examine whether the following are polygons. If any one among them is not, say why?
Solution:
(a) No, because it is not closed,
(b) Yes.
(c) No, because it is not drawn by using line segments.
(d) No, because it is not closed by line segments.
Ex 5.8 Class 6 Maths Question 2.
Name each polygon:
make two more examples of each of these.
Solution:
(a) Quadrilateral
(b) Triangle
(c) Pentagon
(d) Octagon
Two more examples of :
Ex 5.8 Class 6 Maths Question 3.
Draw a rough sketch of a regular hexagon. Connecting any three of its vertices, draw a triangle. Identify the type of the triangle you have drawn.
Solution:
Let ABCDEF be a rough sketch of a regular hexagon.
Join AC
A ∆ ABC is obtained in which AB = BC. Thus,
∆ ABC is an isosceles triangle.
Ex 5.8 Class 6 Maths Question 4.
Draw a rough sketch of a regular octagon.
(Use squared paper if you wish). Draw a rectangle by joining exactly four of the vertices of the octagon.
Solution:
Let ABCDEFGH be a rough sketch of a regular octagon. Join AC, CE, EG and GA to obtain a rectangle ACEG.
Ex 5.8 Class 6 Maths Question 5.
A diagonal is a line segment that joins any two vertices of the polygon and is not a side of the polygon. Draw a rough sketch of a pentagon and draw its diagonals.
Solution:
Let ABCDE be a rough sketch of a pentagon. To draw its diagonals, join AC, AD, BD, DE and CE. Then, AC, AD, BD, BE and CE are its diagonals.
Ex 5.9 Class 6 Maths Question 1.
Match the following:

Give two new examples of each shape.
Solution:
Matching is as under:
(a) → (ii)
(b) → (iv)
(c) → (v)
(d) → (iii)
(e) → (i)
Two new examples of
(a) Cone: Conical tent.
(b) Sphere: Tennis ball, a ball of wool.
(c) Cylinder: Measuring jars, gas cylinder.
(d) Cuboid: Brick, Match box.
(e) Pyramid: Pyramids of Egypt.
Ex 5.9 Class 6 Maths Question 2.
What shape is
(a) Your instruments box?
(b) A brick?
(c) A match box?
(d) A road-roller?
(e) A sweet laddu?
Solution:
(a) Cuboid
(b) Cuboid
(c) Cuboid
(d) Cylinder
(e) Sphere
Related
Discover more from EduGrown School
Subscribe to get the latest posts sent to your email.
